How does temperature affect the performance of an SiPM?

Slawomir Piatek, PhD, Hamamatsu Corporation & New Jersey Institute of Technology
January 4, 2017

Introduction
Quenching resistance
Breakdown voltage
Gain and junction capacitance
Signal shape
Dark counts
Optical crosstalk
Afterpulsing and delayed crosstalk
Photon detection efficiency
Conclusions
References

Introduction

A silicon photomultiplier (SiPM) is a solid-state device capable of detecting single photons due to its very high internal gain (10⁶ – 10⁷). A SiPM is a rectangular matrix of square microcells (10 – 100 µm on the side) numbering from several hundreds to several tens of thousands depending on the model. Each microcell is a series combination of an avalanche photodiode (APD) operating in Geiger mode and a quenching resistor R_Q. All of the microcells are identical and connected in parallel. In a typical operation, a SiPM is reversed biased with the voltage V_BIAS that is a few volts above the breakdown voltage V_BD of the APDs. The difference V_BIAS - V_BD is known as the overvoltage ΔV. Overvoltage is one of the key parameters controlling the operation and opto-electronic characteristics of the detector. In response to light or internally generated charge carriers, a SiPM acts like a current source — the output is a superposition of current pulses produced by the activated, or fired, microcells.

Temperature plays a significant role in the operation of all solid-state devices, including SiPMs. Its varied effects include influencing the concentration of charge carriers in doped and intrinsic semiconductors, thus affecting their resistivity; controlling the phonon spectrum, thus affecting electron-phonon and hole-phonon interactions; and setting the rate of thermal generation of charge carriers, thus affecting the amount of output dark current. This technical note examines and summarizes the present understanding of how changing temperature affects the key opto-electronic characteristics of a SiPM. It relies on published results in peer-reviewed articles and is organized into semi-autonomous sections, each discussing a particular characteristic.

Quenching resistance

R_Q passively terminates Geiger discharge in a microcell. Its value affects the amplitude of a single photoelectron current pulse (often referred to as 1 p.e. pulse or waveform) and, together with the junction capacitance C_J, the recovery time. If the value of R_Q were to fall below a certain threshold, quenching would not have occurred, leaving the microcell in the state of a permanent Geiger discharge and insensitivity to light. The value of R_Q can be deduced from the forward-bias I-V characteristic. As the bias increases, the current eventually becomes linearly proportional to the applied voltage because, as the resistance of the depletion region in the APDs approaches zero, only R_Qs limit the current. Consequently, the slope of the linear section equals N/R_Q, where N is the total number of microcells. Fitting for the slope and knowing N gives R_Q. I-V characteristics obtained at different temperatures yield information on how R_Q varies with T.

Recently, Otte et al. (2016) has performed a comprehensive study of SiPMs from three different manufacturers. Among the many tests, temperature effects within the range –40 °C to +40 °C are prominent. The left panel of Figure 1 shows I-V characteristics for the Hamamatsu S13360-3050CS SiPM, while the right panel shows how R_Q varies with T. The values of R_Q come from linear fits depicted as red lines in the I-V characteristics (left panel).

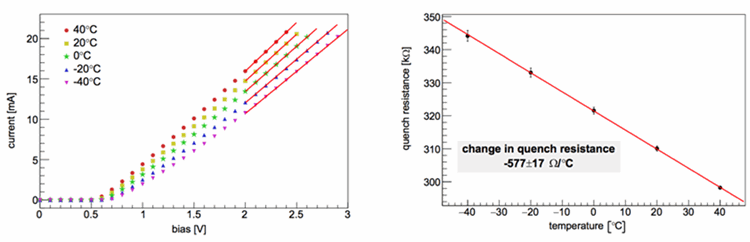
Figure 1. Forward bias I-V characteristics at different temperatures (left panel) and the corresponding values of RQ versus T (right panel). Both panels are from Otte et al. (2016).

A straight line with negative slope is a good fit to the R_Q versus T data. The implied relative change of R_Q with T is about 0.2% per degree Celsius. The negative temperature coefficient implies that R_Q is made from a semiconductor material. Dinu et al. (2015) uses a different approach to measure R_Q. The study finds that the junction capacitance of the investigated SiPMs does not vary with T, therefore, the recovery time of the output current pulses at different temperatures is only affected by R_Q(T). Figure 2 depicts R_Q versus T for two Hamamatsu SiPMs for the temperature range from -175 °C to +55 °C. Note that for the wider range of temperatures, the relationship is no longer linear. Instead, as expected from thermal properties of semiconductors, R_Q is well fit by $a + b T^{1 / 2} e^{(c / T)}$ , where a, b and c are adjustable coefficients. The solid lines in Figure 2 are the best fits.

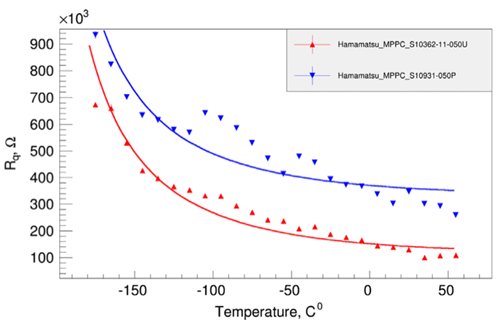
Figure 2. Quenching resistance versus temperature for two Hamamatsu SiPMs. The figure is from Dinu et al. (2015).

Breakdown voltage

Impact ionization of silicon atoms in the avalanche (or high-field) section of the depletion region is the gain mechanism in the constituent APDs of a SiPM. Here, a charge carrier (an electron or a hole) gains energy from the electric field, collides with a silicon atom ionizing it and, thus, creating an electron-hole pair. The probability of impact ionization depends on the strength of the electric field and on temperature. As temperature increases, scattering of charge carriers from the crystal lattice becomes more likely resulting in the net loss of carrier’s energy hindering impact ionization. This can be succinctly stated that the electron and hole ionization rates, α and β, are functions of temperature, both decreasing with T. To compensate the lower ionization rates, the electric field has to be strengthened by increasing the reverse bias. One expects, therefore, that the breakdown voltage V_BD is a function of temperature.

Reverse-bias I-V characteristics at different temperatures can be used to determine V_BD(T). The plot of $\frac{d l n (I)}{d V}$ $\frac{d l n (I)}{d V}$ versus V shows a clearly delineated peak around V_BD with the maximum corresponding to V = V_BD. The left panel of Figure 3 shows a family of such plots for different temperatures, while the right panel depicts the corresponding plot of V_BD versus T. Both figures are for Hamamatsu S13360-3050CS SiPM and are taken from Otte et al. (2016). The figures imply that within the temperature range of the experiments, V_BD increases linearly with T at the rate of about 54 mV/°C — a significant change. Other studies, for example Dinu et al. (2016) and Ferri et al. (2014), also find a linear relationship between V_BD and T for a similar temperature range.

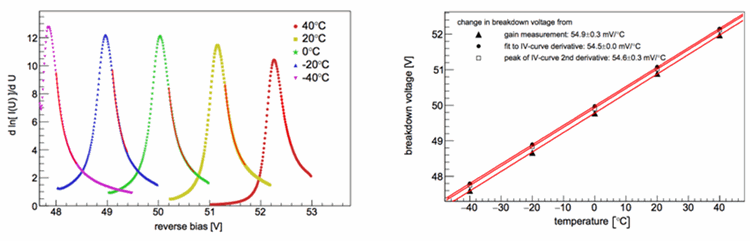
Figure 3. (Left panel) Determination of V_BD from reverse-bias I-V characteristic. The derivative of the natural log of the current with respect to the applied reverse voltage has a maximum at V_BD. (Right panel) The resulting relationship between V_BD and T. Both figures are from Otte et al. (2016).

In another study, Collazuol et al. (2011) uses I-V characteristics to determine VBD for a much wider temperature range (50 K < T < 320 K, or -223 °C < T < 47 °C). Figure 4 shows that for any given small range of temperatures the relationship is approximately linear but over a wider range, it deviates from linearity albeit consistent with theoretical calculation (see, for example, Crowell & Sze (1966)).

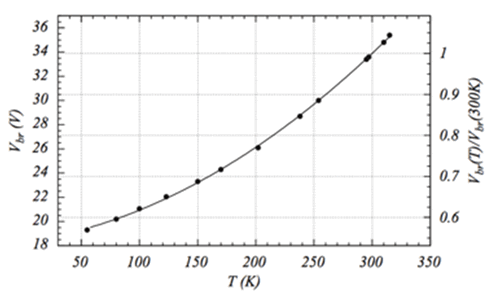
Figure 4. Breakdown voltage versus temperature for a 1 × 1 mm² FBK-IRST SiPM type T6-V1-PD with 625 40 × 40 µm² microcells. The figure is from Collazuol et al. (2011).

The gain µ of a SiPM equals the number of electrons that contribute to the 1 p.e. output current pulse i(t). Therefore,

Equation 1

μ = \int_{0}^{\infty} i (t) d t \approx \frac{(V_{B I A S} - V_{B D}) C_{J}}{e} = \frac{∆ V C_{J}}{e}

where C_J is the junction capacitance of a single APD and e is the elementary charge. Stability of the gain under changing ambient temperature is a common requirement in practical applications. This can be accomplished by adjusting V_BIAS to compensate changes of V_BD and, potentially, C_J with T.

Gain and junction capacitance

As discussed above, the gain of a SiPM can be measured by integrating 1 p.e. waveforms. Doing so as a function of V_BIAS and for different temperatures offers another method of determining V_BD and a way of finding how C_J depends on T. The left panel of Figure 5 shows plots of µ versus V_BIAS at several temperatures, while the right panel shows the corresponding plot of C_J versus T for Hamamatsu S13360-3050CS SiPM. Both figures are from Otte et al. (2016). For a given temperature, the plot of µ versus V_BIAS is well fit by a straight line, implying no dependence of C_J on V_BIAS. The value of V_BD equals V_BIAS when µ = 1, which is essentially the X intercept. The slope equals C_J/e, allowing an easy determination of C_J. The right panel of Figure 5 shows that C_J has a statistically weak dependence on T for this particular SiPM; however, other SiPMs in the study have a more significant dependence, as illustrated in Figure 6 for SensL J-series 30035 SiPM. The straight lines at different temperatures are parallel to each other implying that µ is independent of T provided that ∆V remains the same.

Click here to read more about the effects of temperature on the gain of an SiPM.

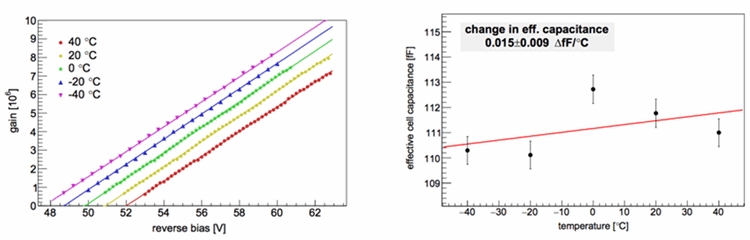
Figure 5. (Left panel) Gain versus bias voltage for several temperatures and (right panel) junction capacitance versus temperature for Hamamatsu S13360-3050CS SiPM. Both figures are from Otte et al. (2016).

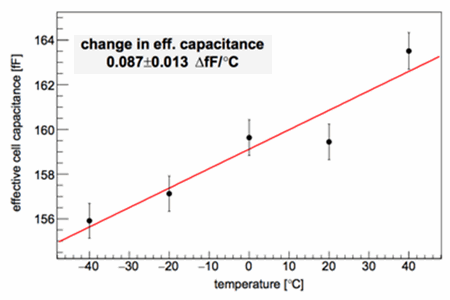
Figure 6. Junction capacitance versus temperature for SensL J-series 30035 SiPM. The figure is from Otte et al. (2016).

Signal shape

The shape of the output current pulse depends on the values of R_Q and C_J (and also on the bandwidth of the detection system and load resistance). Since R_Q does and C_J may vary with T, the shape too will be a function of T, as illustrated in Figure 7 (adapted from Dinu et al. 2015). The figure shows that a higher T makes a SiPM "faster" (shorter recovery time), which is highly desirable in some applications. However, the tradeoff is an increase in dark counts whose consequence is a higher minimum detectable power, or lower sensitivity.

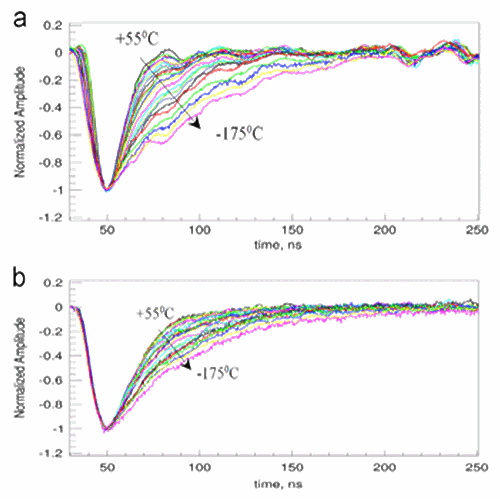
Figure 7. Normalized output averaged signals at different temperatures for (top panel) 1 × 1 mm² and (bottom panel) 3 × 3 mm² Hamamatsu SiPMs. The bandwidth of the detection system is 20 MHz. The figure is from Dinu et al. (2015).

Dark counts

A thermally generated (or due to quantum tunneling) charge carrier may enter (or be generated where) the high field region of an APD and trigger Geiger discharge. This possibility is known as a dark count. It results in an output that is indistinguishable from that resulting from absorption of a photon. Counting the number of the output waveforms with amplitude greater than one half of that for 1 p.e. waveform in a time interval τ gives the rate in Hertz (counted number divided by τ). The rate is a function of ΔV and T, as shown in the left panel of Figure 8. Note that the abscissa is actually the relative overvoltage:

Equation 2

∆ V_{r} \equiv \frac{(V_{B I A S} - V_{B D})}{V_{B D}} = \frac{∆ V}{V_{B D}}

The right panel shows how the rate changes with T relative to the rate at 40 °C for a fixed value of ΔV_r. The rate doubles every 5.3 °C for this particular SiPM.

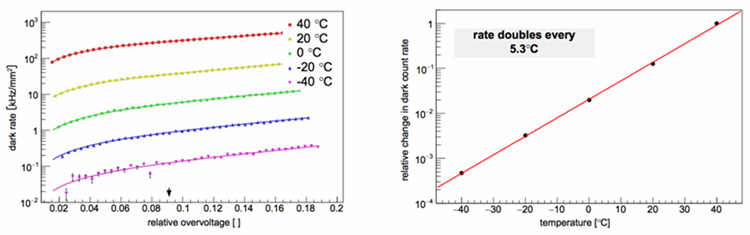
Figure 8. (Left panel) Dark count rate versus relative overvoltage for several temperatures and (right panel) relative change in dark count rate versus temperature. The figures are for Hamamatsu S13360-3050CS SiPM and adopted from Otte et al. (2016).

Noteworthy is a measurement of dark count rate as a function of T for a fixed overvoltage (1.5 V) by Collazuol et al. (2011), shown in Figure 9. The wider temperature range allowed the study to identify distinct sources of dark counts. Shockley-Read-Hall generation enhanced by trap-assisted tunneling is mostly responsible for dark counts in the region labeled a), whereas band-to-band tunneling dominates the region b). The study notes that region c) is still under investigation.

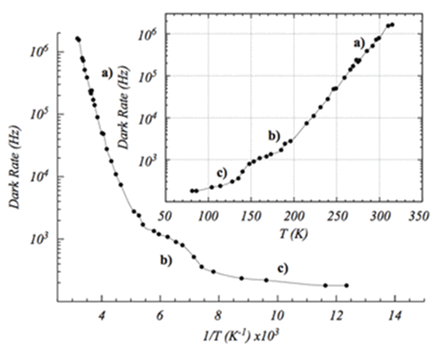
Figure 9. Dark count rate versus temperature for the same SiPM as in Figure 4. The figure is from Collazuol et al. (2011).

Optical crosstalk

Optical crosstalk is a type of correlated noise ubiquitous in SiPMs. It comes in two types: direct and delayed. When a microcell undergoes Geiger discharge, it emits about 2.9 x 10^-5 photons per electron that can be reabsorbed by silicon (Lacaita et al. 1993). The total number of emitted photons is $2.9 \times 10^{- 5} μ = 3 \times 10^{- 5} \frac{∆ V C_{J}}{e}$ , or about 29 for µ = 10⁶. A direct crosstalk occurs when a photon from the primary discharge moves either directly, or after a reflection, to the avalanche region of a neighboring microcell and triggers there a secondary discharge. The two resulting current pulses are nearly simultaneous producing a 2 p.e. output waveform. However, if the emitted photon is absorbed outside of the avalanche region but within the depletion region, one of the charge carriers can drift into the avalanche region and triggers a secondary discharge. The two resulting current pulses are spaced chronologically by the drift time. This is delayed crosstalk, which can resemble afterpulsing (discussed in the next section). The following discussion applies only to direct crosstalk.

The probability of optical crosstalk P_CT is expected to increase with ΔV because the number of the emitted photons is linearly proportional to ΔV and because the probability of Geiger discharge P_G increases with ΔV. The dependence of P_CT on T for a fixed ΔV will be mostly determined by the dependence C_J with T (since C_J affects the gain and, therefore, the number of emitted photons). Figure 10 is a plot of P_CT as a function of ΔV_r at several temperatures for Hamamatsu S13360-3050CS SiPM. For a given ΔV_r, there is only a weak dependence of P_CT on T, reflecting a weak trend seen in Figure 5. In contrast, Collazuol et al. (2011) finds no evidence that P_CT depends on T, which is possible if C_J of the investigated SiPM is independent of T.

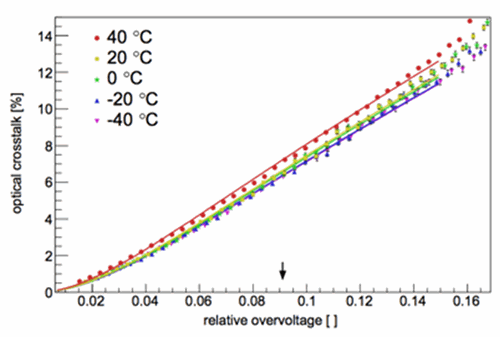
Figure 10. Probability of direct optical crosstalk as a function of relative overvoltage at several temperatures. The figure is from Otte et al. (2016).

Afterpulsing and delayed crosstalk

Impurities in silicon and crystal lattice defects can act as charge traps. A trap can capture a charge carrier created during an avalanche and then release it at a later time. If the release occurs during the recovery, the number of electrons produced in the afterpulse is less than µ and the resulting afterpulse waveform partly overlaps in time the primary; the output is their superposition. In contrast, if a trap releases charge after the complete recovery, the resulting afterpulse waveform does not overlap the primary and it is indistinguishable in shape from a 1 p.e. waveform.

Delayed crosstalk has a somewhat different signature. Here, the secondary waveform has the same amount of charge as the primary. If the drift time is less than the recovery time, the output consists of a superposition of two 1 p.e. waveforms, whereas if it is longer, two separate 1 p.e. waveforms result. The characteristic times of afterpulsing and delayed crosstalk are expected to be different because the physical processes governing them are different. Therefore, one can estimate their rates, or probabilities, from a histogram of time differences between consecutive pulses (for more details of this rather difficult measurement see Otte et al. (2016)).

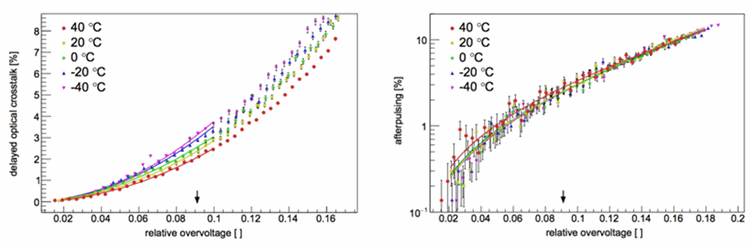
Figure 11. Plots of probability of optical crosstalk (left panel) and afterpulsing (right panel) versus relative overvoltage at several temperatures for Hamamatsu S13360-3050CS SiPM. Both plots are from Otte et al. (2016).

The plot in the left panel of Figure 11 shows how the probability of delayed optical crosstalk depends on relative overvoltage at several temperatures. As expected, the probability increases with ΔV_r, though for a nominal ΔV_r (marked with an arrow), it amounts to only about 2%. For fixed ΔV_r, the probability increases with decreasing T (surprisingly, other SiPMs in the study show the opposite effect). The plot of afterpulsing probability as a function of ΔV_r, shown in the right panel of Figure 11, does not exhibit any significant dependence on T. This is surprising because the trapping time constant is known to decrease exponentially with T, therefore, afterpulsing probability should decline with T. The probabilities of afterpulsing for the other two SiPMs investigated in the study show the opposite dependence with T. These surprising and contradictory results may have to be clarified with additional future testing. Otte et al. (2016) does note that the afterpulsing data may have been contaminated with delayed crosstalk.

Photon detection efficiency

Photon detection efficiency (PDE) is a probability that a SiPM produces an output signal in response to an incident photon. PDE is defined as a product of a geometrical fill factor, quantum efficiency (QE), and probability of Geiger discharge P_G. PDE is expected to depend strongly on ΔV (through P_G) and may show some dependence on T. The reasons for the latter are: 1. The band gap energy is a function of temperature, causing QE = QE(T) especially for the wavelengths close to the threshold wavelength; 2. Carrier mobility increases with T, thus P_G may increase with T for fixed overvoltage; 3. Free carrier concentration depends on T, thus affecting all of the parameters that depend on the concentrations. There is very little reliable research investigating how T affects PDE. Near room temperatures, the effect is likely to be negligible but for a wider range, this may not be so, as indicated by Figure 12 from Collazuol et al. (2011). As noted by the study, more work is needed to fully understand PDE(T).

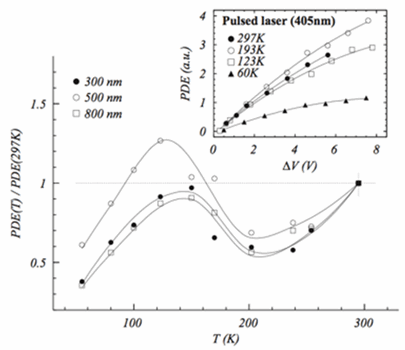
Figure 12. Normalized photon detection efficiency as a function of temperature for an overvoltage of 2V and three wavelengths (300 nm, 500 nm, and 800 nm) for 1 × 1 mm² FBK-IRST SiPM type T6-V1-PD. The insert shows PDE as a function of overvoltage at four different temperatures.

Conclusions

Changing temperature can have a significant impact on the operation of a SiPM. Two strong dependencies on T are the value of the breakdown voltage and the dark count rate. For a stable gain, it is necessary that the ambient temperature is constant or that VBIAS is adjusted to keep the overvoltage fixed. The latter requires using a temperature-compensation circuitry. Higher dark count rate increases the minimum detectable power. In low-light applications, it may be necessary to cool the SiPM to increase its sensitivity.

References

G. Collazuol, et al., Nuclear Instruments and Methods in Physics Research Section A 628 (2011) 389.
Crowell, C. R., & Sze, S. M., Appl. Phys. Lett. 9 (1966) 242
Dinu, N., Nagai, A., & Para, A. "Studies of MPPC detectors down to cryogenic temperatures," Nuclear Inst. And Methods in Physics Research, A, 787, (2015), 275-279
Dinu, N., Nagai, A., & Para, A. "Breakdown voltage and triggering probability of SiPM from IV curves at different temperatures," Nuclear Inst. And Methods in Physics Research, A, 2016, in press
Ferri, A., Acerbi, F., Gola, G., Paternoster, C, Piemonte, C, & Zorzi, N. "A comprehensive study of temperature stability of Silicon Photomultiplier," JINST, 2014
A. Lacaita, M. Ghioni, F. Zappa, G. Ripamonti, S. Cova, Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment 326 (1993) 290.
Otte, A. N, Garcia, D., Nguyen, T., Purushotham, D. "Characterization of Three High Efficiency and Blue Sensitive Silicon Photomultipliers," Nuclear Inst. And Methods in Physics Research, A, 2016, in press.
Piatek, S., "Effects of temperature on the gain of a SiPM," www.hamamatsu.com, published December 2016.

How does temperature affect the performance of an SiPM?

Introduction

Quenching resistance

Breakdown voltage

Gain and junction capacitance

Signal shape

Dark counts

Optical crosstalk

Afterpulsing and delayed crosstalk

Photon detection efficiency

Conclusions

References

1. What are cookies?

2. What are the different types of cookies?

3. How do we use cookies?

4. Which cookies do we use?

5. What are Internet tags and how do we use them with cookies?

6. Analytics and Advertisement Cookies